Introduction

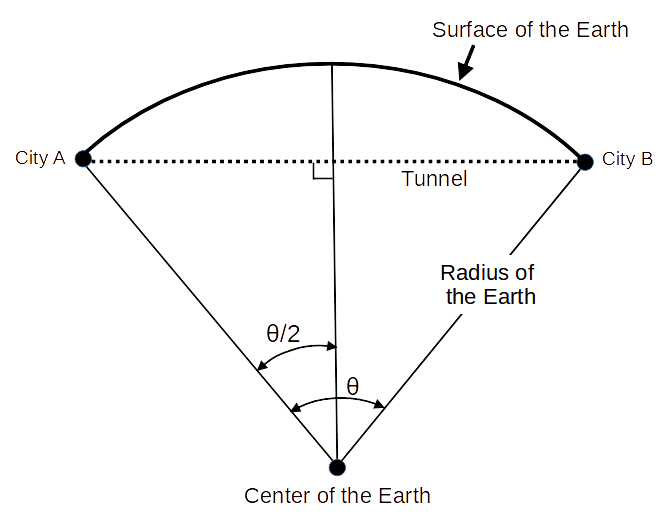
The shortest distance (path) between cities (A and B) on the surface of the earth is a great-circle distance. Instead, what if we drilled a tunnel directly between city A and city B?

Create an interactive program to:

1. let the user select city A and city B from a list of cities
2. convert the latitude and longitude values from degrees to radians
3. calculate distances and angles
4. display distances and angles
5. loop

Great-Circle

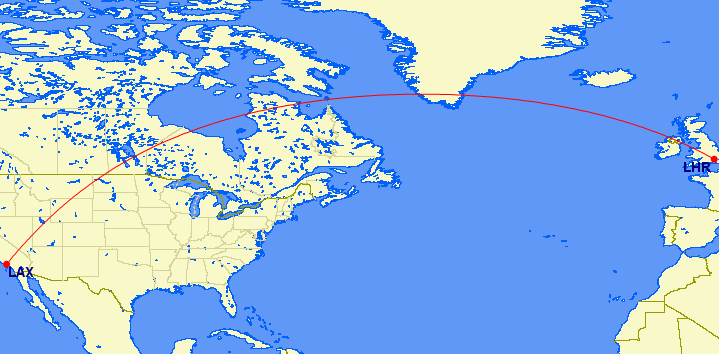
Note: The great-circle route is the shortest course between two points on the surface of a sphere. This is why when you fly from Los Angles to London, You leave LA flying north east and fly over Greenland instead flying directly east.

Great-circle distance (Wikipedia)

List of City Latitude/Longitude

Note: Add your own cities to the list.

City	Latitude/Longitude DDD° MM′ SS.S″
Athens, Greece	37°58'N, 23°43'E
Beijing, China	39°55'N, 116°25'E
Royal Observatory Greenwich, England	51.4769°N, 0.0005°E
London, England	51°32'N, 0°5'W
Los Angeles, California	34°3'N, 118°15'W
Moose Jaw, Saskatchewan, Canada	50°7'W, 105°31'N
Moscow, Russia	55°45'N, 37°36'E
New York City, New York	40.7128°N, 74.0060°W
Nome, Alaska	64°25'N, 165°30'W
Paris, France	48°48'N, 2°20'E
Tokyo, Japan	35°40'N, 139°45'E
San Francisco, California	37°47'N, 122°26'W
Washington, D.C.	38°53'N, 77°02'W
Wellington, New Zealand	41°17'S, 174°47'E

Definition: The great-circle distance is the distance along a great circle. It is the shortest distance between two points on the surface of a sphere.

Code

Calculate Great-Circle Distance
Calculate Great-Circle Angle
Calculate Center of the Earth to the Center of the Tunnel Distance

For the following projects assume the Earth is a perfect sphere. It is not.

Project #0

What is its actual shape of the Earth?

Hint: The Earth is an oblate ...

Project #1

What is the great-circle distance on earth for:

• one degree
• one minute
• one second

To be consistent, use the values in the "Useful Information" table below.

Note: Project #1 does not require any fancy math. Just division.

Project #2

How much shorter would the tunnel be compared to the great-circle distance?

Project #3

What is the deepest distance between the tunnel and the surface of the earth?

Useful Information

1. The distance from the center of the earth to point A and point B are the same distance. They are two side of an isosceles triangle.
   Definition: An isosceles triangle is a triangle that has two sides of equal length.



2. 3.14159	pi
   24875.11	circumference of Earth in miles based on global average radius
   40030.14	circumference of Earth in kilometers based on global average radius
   3959	radius of Earth in miles global average (wikipedia)
   6,371	radius of Earth in kilometers global average (wikipedia)
   5280	feet per mile
   3.28084	feet per meter
   1.60934	kilometers per mile
   1000	meters per kilometer
   360	degrees in a circle
   60	minutes per degree
   60	seconds per minute




3. Sine of acute angle	the length of the side opposite the angle divided by the length of the longest side (the hypotenuse)
   Cosine of acute angle	the length of the side adjacent to the angle divided by the length of the longest side (the hypotenuse)

   Note: Acute angles measure less than 90 degrees. Right angles measure 90 degrees. Obtuse angles measure more than 90 degrees.